Home 
  OpticsPOD
  What's New 
  Rays & Shadows
  Water Droplets
  Rainbows
    Not a rainbow!
    Primary Bow
    Secondary Bow
    A's Dark Band
    Other Orders
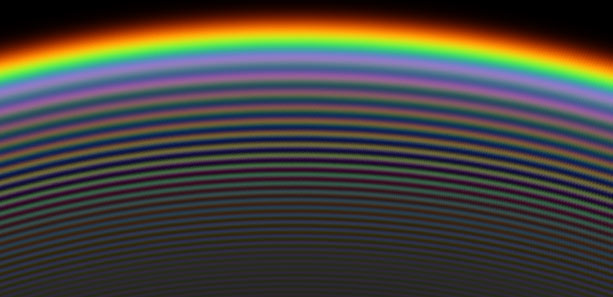
    Supernumeraries
    Red Bows
    Rainbow Wheel
    Dew Bow
    Sea Spray Bow
    Glass Bead Bows
    Reflection Bows
    Twinned Bows
    Cloud Bows
    Moon Bows
    Image Gallery
    Simulators
      BowSim
      AirySim
  Ice Halos
  High Atmosphere
  Links & Resources
  Search - Index



                 

123456789012345678
   AirySim 

 
    Bow from 0.75mm diameter drops illuminated by a distant point source. Supernumeraries like these are not seen in nature because they are blurred by the finite angular size of the sun and variations in drop size. AirySim simulation.
   		     
  When a plane light wave interacts with a water drop the outgoing wave after a single internal reflection is curved. If the shape of the wave is somehow known then phase differences along it can be calculated and thus the intensity of the resulting rainbow and its supernumeraries. The English Astronomer Royal, George Biddell Airy (1801-1892), approximated the scattered wavefront shape with a cubic form and developed an analytic expression for the rainbow intensities in terms of what are now called Airy integrals or functions. Airy's theory gives satisfactory predictions of the observable features of white light rainbows(1) and is computationally very considerably faster than the exact predictions of Mie theory.

AirySim precomputes and stores Airy functions for a whole range of arguments using an ascending series expansion(2). To compute a rainbow for a particular drop size, wavelength and refractive index(3), appropriate values of the Airy functions for each scattering angle are derived by interpolation of the stored values or, where necessary, additional direct computation. White light rainbows are obtained by repeating the calculation for closely spaced wavelengths between 380 and 700 nm and summing the intensities at each angle after weighting them by a spectral solar radiance(4).

When simulations from non monodisperse droplets are required AirySim uses a droplet population function normally distributed in radius. All the above calculations have to be repeated for the different droplet radii and the intensities summed.

Rainbows from the sun rather than plane parallel light are derived by convolving the angular intensities with a disk intensity function.

Representation of colours is always problematic. AirySim uses the CIE and Bruton(5) colour models written for IRIS.
   
Regrettably, AirySim is not available for download. The intentions were good bit I did not have the time to construct the necessary user friendly interface.
(1)  Lee, R. L., "Mie theory, Airy theory, and the natural rainbow," Applied Optics 37, 1506-1519 (1998).
http://www.usna.edu/Users/oceano/raylee/papers/RLee_papers.html
  
(2)  Abramowitz M. & Stegun I.A., Handbook of Mathematical Functions, Dover.
  
(3)  Water refractive indices are from IAPWS (International Association for the Properties of Water and Steam) which are in turn based on P. Schiebener, J. Straub, J. M. H. L. Sengers, J. S. Gallagher, "Refractive index of water and steam as function of wavelength, temperature and density," J. Phys. Ch. R.,19, 677-717, (1990).
(4)  Spectral solar radiances were those used by Raymond Lee(1) and kindly supplied by him in detailed tabular form.
  
(5)  Dan Bruton of Stephen F. Austin State University, "Color Science", http://www.physics.sfasu.edu/astro/color.html