toptics
A
Highlights
Atomic Fogbow
Not the familiar fogbow that graces water droplet laden skies. Instead a scattering pattern generated by potassium atoms colliding in a high vacuum apparatus. The atoms behave like waves that interfere. Waves of quantum mechanical probability if you like. The resemblance to a fogbow is more than coincidental for some, not all, of the physics is similar.
All images ©Les Cowley
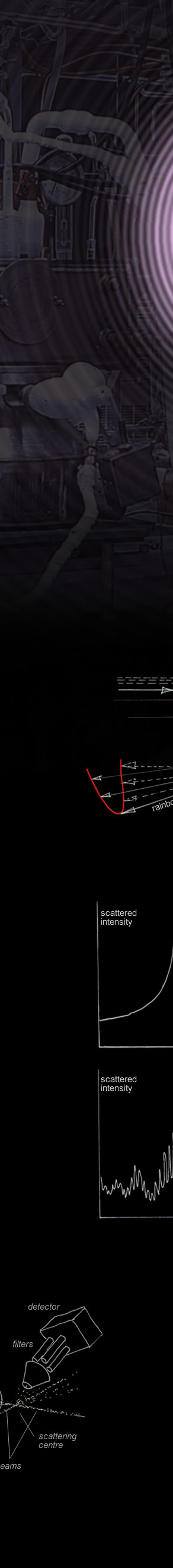
A rainbow spike for elastic atom collisions predicted by classical mechanics. Like an optical rainbow, the angles within the rainbow are bright and the outside dark.
Not all interatomic forces or collision energies give a rainbow.
Atomic fogbows and rainbows
Billiard balls strike with a hard 'clack'. Before that they are quite unaware of each other.
Atoms are different. At large distances they attract each another. Fluctuating electron distributions in one induce charge separation in the other - result, attraction. As they approach the attraction switches to stronger and stronger repulsion. The repulsion is from the two positively charged nuclei plus a quantum effect – Pauli exclusion repulsion. Pauli exclusion is the same force that stops neutron stars collapsing.
We might try classical mechanics to predict an atom's path though this mixed attractive/repulsive force field (3). Some trajectories are at right. The path starting at ‘a’ is hardly affected. As the atom skims closer to the force centre it deflects more. Inside ‘b’, repulsive forces dominate. At 'c' the atom deflects strongly in the opposite direction.
At ‘b’ the deflection turns around and paths cluster there - Just as light rays do to form a rainbow! Classically, we would see a large spike in the scattered atom intensity at a certain angle – an atomic rainbow.
What actually happens is more interesting. At everyday thermal energies, classical mechanics doesn't work and we must instead use quantum mechanics.
A plane wave crossing the central force field replaces the atom particle. The plane wave scatters to make an outgoing spherical wave (4). A probability wave perhaps. The classical atomic rainbow spike transforms into a series of oscillations analogous to a fogbow’s primary and inner supernumeraries.
Those oscillations are a rich source of information about the interaction.
Meanwhile, back in the lab
Someone once said that molecular beam research was like peering hopefully (or hopelessly!) at the ragged edge of invisibility.
The source atoms must be collimated by slits into strongly directional beams. The beams are filtered to pass only narrow velocity ranges. Other properties like mass, rotational state or electronic spin might be selected too. All that gives weak final beams and few collisions where they cross. Detectors need further filtering and have to count individual atoms or molecules. Alternatively, some methods detect instead the light emitted during the collisions.
To detect the full detail of theoretical predictions would be marvelous. Usually only major features might be discerned.
Yet what is seen tells directly of the forces between atoms and molecules. It tells how they collide and exchange energy. It reveals intimate step by step details of how some react to birth new molecules.
For fascinating accounts of early research on molecular beams and chemical dynamics see the Nobel lectures of
Dudley Herschbach and John Polanyi
Fogbows and atomic scattering
A - A fogbow made by 10 micron dia. water droplets. It has prominent supernumeraries and a central glory.
B - The same fogbow in monochromatic light. Mie predictions have strong angular fluctuations shifting rapidly as the wavelength changes.
C - A scattering pattern from colliding atoms
Atoms and molecules collide incessantly in the air. But to glean knowledge from collisions they need to be made (footnote 1) under far more controlled conditions.
One way is via crossed molecular beams. Two narrow beams of atoms or molecules are directed at each other in a high vacuum chamber (2). The atoms make a single collision and we measure the way they scatter apart.
The scattering pattern above is a theoretical prediction for atoms hitting each other at 1 km/s. That is an ordinary thermal energy. Measurements of scattering patterns reveal fine details of the forces between atoms and how they interact.
The small print
1) Atoms in the air or gases collide furiously at a whole range of speeds and directions. Properties that depend on collisions like viscosity or chemical reaction rates are huge averages over this range and measuring them tell us little about the individual elementary collisions and fundamental processes occurring.
2) The best possible vacuum is needed to avoid secondary collisions with the chamber's residual background gas. Not easily achieved and stages of differential pumping are employed.
3) This text talks mostly about 'elastic' collisions, i.e. those where there is no change in total translational energy. Many other collision interactions occur where there are changes in translational, electronic, vibrational and rotational energy not to mention spin and exchange of atoms between the colliding groups - chemical reaction.
The 'two body' problem of colliding atoms can be exactly replaced by that of a single body of reduced mass passing across a potential energy surface V(r). V(r) is that existing between the two original atoms with r the interatomic distance. The trajectories shown here are in that spirit. Gravitational attraction is similarly treated but gravity falls off slowly at the inverse square of distance. Atomic forces are shorter range and vary as the inverse sixth to twelfth power.
4) Briefly, radial wave differential equations are solved for up to 1000 values of the collision angular momentum quantum number 'L'. For each 'L' the phase shift is derived for waves with and without the potential V(r) acting. All the collision information is in the phase shifts. Scattering cross sections for a range of angles are then computed using a 'partial wave' method first derived by Lord Rayleigh for the scattering of sound waves through balloons of different gases!
An equivalent quantum mechanics prediction. The atoms behave as waves. The classical rainbow spike is replaced by broader peaks, inner supernumeraries and a superimposed higher angular frequency oscillatory structure.
Experimental measurements on the structure enable determination of the interatomic forces.
At left: Rays through a raindrop have a minimum deflection (turning point) where they cluster to form a rainbow.
Below: Classical atom collision trajectories have a similar turning point. This too forms a 'rainbow'.