 |
 |
 |
 |
 |
 |
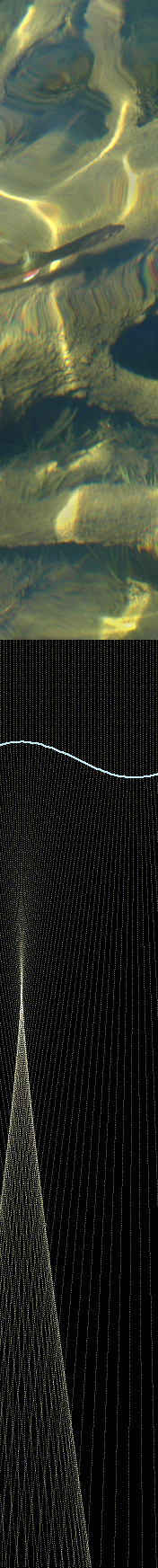
Trout and Optical Catastrophes
Imaged by Andrew Kirk. "I was photographing rapidly moving caustics and a dead tree on the bottom of a
wide stream, when a trout floated into the frame. I then began to attempt to
catch the caustics as they crossed the trout. The distortions of the trout were
too rapid to see, but the camera caught them!" ©Andrew Kirk, shown with permission. |
 |
 |
 |
 |
 |
 |

| About - Submit | Optics Picture of the Day | Galleries | Previous | Next | Today |
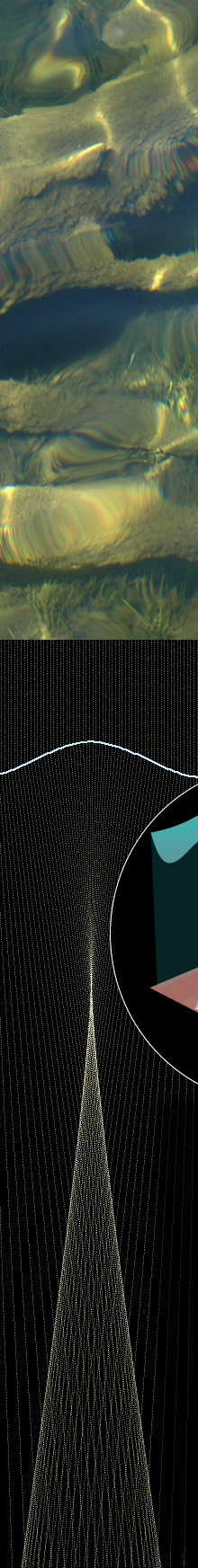
Refraction by the wavy water surface distorts the trout and produces bright lines on the stream bed, ‘caustics’. |
| Top: Light rays crossing and bunching together form a bright caustic surface (a fold caustic in this example). More profoundly, a caustic marks a topological discontinuity (a catastrophe) in ray behaviour. Upper right: Caustic sheets below a wavy surface illuminated by an overhead sun. For each wave crest there are paired sheets (fold caustics) joined at top by a cusp caustic. Where the fold caustics intercept a stream bed they give a bright line. Background image: An accurate computation for vertical rays refracted by a wavy water surface. Three rays from the wave crests form the cusps. From elsewhere on the wave, two rays intersect on each point of the downward going fold caustic sheets. Rays from near the wave midpoints where the surface has an inflexion point form the very distant caustics. The waves were made high amplitude to fit the caustics onto the page. We usually see caustics from gentler surfaces and then the pairing of caustics with brighter regions between them is less obvious. |